Elevation model (Water Overlay): Difference between revisions
No edit summary |
mNo edit summary |
||
| Line 1: | Line 1: | ||
The elevation model is : | |||
Rasterization of the height sectors | * Rasterization of the height sectors | ||
Piecewise linear reconstruction of the bottom | * Piecewise linear reconstruction of the bottom | ||
===Piecewise linear reconstruction of the bottom=== | |||
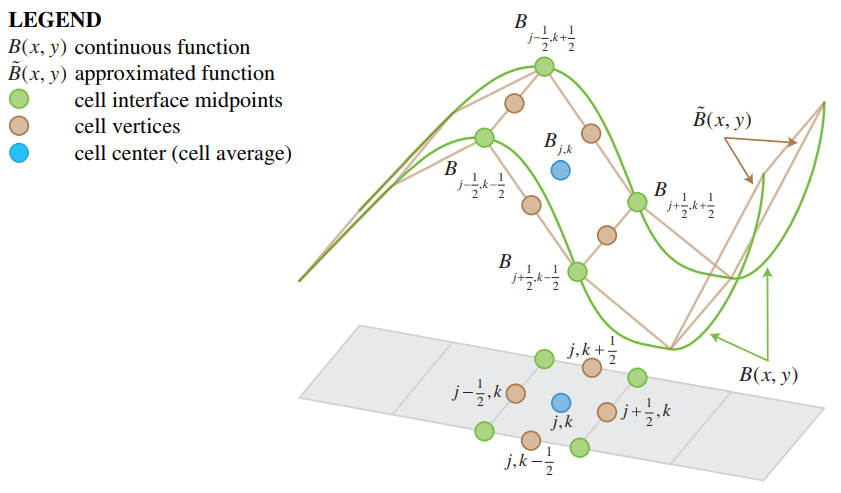
The implementation of the Water Module is based on second-order semi-discrete central-upwind scheme by Kurganov and Petrova (2007)<ref name="Kurganov2"/>. The [[terrain height (Water Overlay)|surface elevation]], also named bottom in the paper, is slightly adjusted to support the scheme to become ''well balanced and positivity preserving''. The process of adjusting the original surface elevation is called ''piecewise linear reconstruction of the bottom''. | |||
[[File:piecewisereconstruction_1d.png|left|thumb|400px|1D linear piecewise reconstruction.Source: Kurganov and Petrova (2007)<ref name="Kurganov2" />]]{{clear}} | |||
The first requirement the scheme to become ''well balanced and positivity preserving'' is to ensure that each grid cell has a constant linear slope in both the x- and y- direction. Secondly the end points of the slope should meet in the center of the cell's edges. This ensures that the bottom is continuous along cells in the x- and y- direction. Thirdly, the linear slope in the x- and y-direction within a cell should meet in a single center point. | The first requirement the scheme to become ''well balanced and positivity preserving'' is to ensure that each grid cell has a constant linear slope in both the x- and y- direction. Secondly the end points of the slope should meet in the center of the cell's edges. This ensures that the bottom is continuous along cells in the x- and y- direction. Thirdly, the linear slope in the x- and y-direction within a cell should meet in a single center point. | ||
| Line 22: | Line 18: | ||
The only down sight is that the new center point might have been placed height or lower in case the terrain 's slope was originally not linear within the cell. | The only down sight is that the new center point might have been placed height or lower in case the terrain 's slope was originally not linear within the cell. | ||
<ul> | <ul> | ||
<li style="display:inline-block">[[File:Inundation overlay 04 HWP(2).PNG|thumb| | <li style="display: inline-block;">[[File:Inundation overlay 04 HWP(2).PNG|left|thumb|400px|2D linear piecewise reconstruction. Source: Horváth et al. (2014)<ref name="Horvath"/>]]</li> | ||
<li style="display:inline-block">[[File:Inundation overlay 04 HWP(1).PNG|thumb| | <li style="display: inline-block;">[[File:Inundation overlay 04 HWP(1).PNG|left|thumb|400px|Application of reconstructed elevation Source: Horváth et al. (2014)<ref name="Horvath"/>]]</li> | ||
</ul> | </ul> | ||
===Notes=== | ===Notes=== | ||
* The smaller the grid size, the closer the bottom reconstruction will approximate the original surface elevation. | * The smaller the [[grid cell size|grid size]], the closer the bottom reconstruction will approximate the original surface elevation. | ||
==References== | ==References== | ||
| Line 36: | Line 31: | ||
</references> | </references> | ||
{{WaterOverlay nav}} | |||
{{Water Module buttons}} | {{Water Module buttons}} | ||
Revision as of 13:38, 18 June 2019
The elevation model is :
- Rasterization of the height sectors
- Piecewise linear reconstruction of the bottom
Piecewise linear reconstruction of the bottom
The implementation of the Water Module is based on second-order semi-discrete central-upwind scheme by Kurganov and Petrova (2007)[1]. The surface elevation, also named bottom in the paper, is slightly adjusted to support the scheme to become well balanced and positivity preserving. The process of adjusting the original surface elevation is called piecewise linear reconstruction of the bottom.

The first requirement the scheme to become well balanced and positivity preserving is to ensure that each grid cell has a constant linear slope in both the x- and y- direction. Secondly the end points of the slope should meet in the center of the cell's edges. This ensures that the bottom is continuous along cells in the x- and y- direction. Thirdly, the linear slope in the x- and y-direction within a cell should meet in a single center point.
To fulfill these requirements, the following steps are used:
- Pick or calculate the height points for the 4 corners of the cell.
- Form a rectangle with the 4 corners and calculate the centers of these edges. (These are the points that have to meet for continuity)
- Calculate a new center point based on the 4 edge center points.
Given that the adjacent cells share the same corner points, and thus share an edge center point, the bottom will be continuous in the x and y direction. Furthermore, the cell has an linear slope in both the x- and y-direction. The only down sight is that the new center point might have been placed height or lower in case the terrain 's slope was originally not linear within the cell.
2D linear piecewise reconstruction. Source: Horváth et al. (2014)[2] Application of reconstructed elevation Source: Horváth et al. (2014)[2]
Notes
- The smaller the grid size, the closer the bottom reconstruction will approximate the original surface elevation.
References
- ↑ 1.0 1.1 Kurganov A, Petrova G (2007) ∙ A Second-Order Well-Balanced Positivity Preserving Central-Upwind Scheme for the Saint-Venant System ∙ found at: http://www.math.tamu.edu/~gpetrova/KPSV.pdf (last visited 2018-06-29)
- ↑ 2.0 2.1 Zsolt Horváth, Jürgen Waser, Rui A. P. Perdigão, Artem Konev and Günter Blöschl (2014) ∙ A two-dimensional numerical scheme of dry/wet fronts for the Saint-Venant system of shallow water equations ∙ found at: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.700.7977&rep=rep1&type=pdf ∙ http://visdom.at/media/pdf/publications/Poster.pdf ∙ (last visited 2018-06-29)